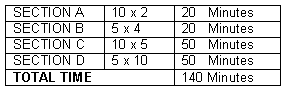Many
students, even some really intelligent and talented ones, have a
strange enemy. They often find it difficult to finish the paper within
the allotted time. They are forced to leave a few questions just because
they run out of time and often it has been found that the questions
they leave are those which they otherwise could do very easily. It can
be very disappointing if you are forced to skip such easy questions.
But how can you avoid a situation like this? Many people suggest a single tablet for this "Time management". But how to manage time and how to stop it from running out is a difficult proposition, especially for an average 14-15-year-old tenth grader.
Here are certain tips by experts from to help you out to finish your paper well before time.
1. Understand your examThe most important thing is to understand the examination you are about to take. In the class X mathematics paper, there are 30 questions in four sections A, B, C and D and we have 180 minutes to answer these questions. Here, a rough calculation is that we get about six minutes to answer a question. But that is not the fact.
The question paper contains 'very short answer' type, 'short answer' type and 'long answer' type questions and the time requirement for each type is different. An ideal allotment for the four sections is as shown below:
Additionally,
you must practice the habit of finishing samples papers in 140-150
minutes. This will help you simulate and exercise examination pressures
better.
But how can you avoid a situation like this? Many people suggest a single tablet for this "Time management". But how to manage time and how to stop it from running out is a difficult proposition, especially for an average 14-15-year-old tenth grader.
Here are certain tips by experts from to help you out to finish your paper well before time.
1. Understand your examThe most important thing is to understand the examination you are about to take. In the class X mathematics paper, there are 30 questions in four sections A, B, C and D and we have 180 minutes to answer these questions. Here, a rough calculation is that we get about six minutes to answer a question. But that is not the fact.
The question paper contains 'very short answer' type, 'short answer' type and 'long answer' type questions and the time requirement for each type is different. An ideal allotment for the four sections is as shown below:
2. Use the first 15 minutes effectively
You
get a good 15 minutes in the beginning to read the question paper --
use this time to do just that, READ. Read all the 30 questions in 15
minutes. While reading, mark the questions into categories viz easy,
manageable and tough. This is done to have an overall idea about the
questions and make a rough plan.
3. Don't worry about the tough ones
The
moment you find that there are a few tough questions; it is natural
that you start worrying about them. This is not required and will only
harm your performance.
The
fact is that they may look a bit tough on the surface, but when you
actually work on them you will find most of them to be much easier than
they seemed. So be happy about the easy ones and don't get unduly
worried about the tougher lot.
4. Prioritise your attempt
Always
attempt the easy questions first and then move on to the manageable
ones and ensure that you complete them before taking on the difficult
ones. This will ensure that you are not leaving any question that you
know.
Once you successfully attempt all the easy and manageable questions, your confidence will grow and you will be
mentally ready to take on the more challenging questions.
5. Ensure speed and accuracy
Use
quicker methods in calculations to ensure that you are not wasting time
and your answers are correct. Mostly, we take a lot of time to solve a
problem if we happen to make some error in the process.
For
example, if you make an error in the sign of a term (+/-), you may not
be able to solve questions involving quadratic equations or linear
equations. Therefore avoiding silly mistakes is very important to save time.
6. Keep an eye on your watch
Keeping
an eye on your watch is of course not to increase your stress. This is
just to see that you are broadly adhering to the time allocation we
discussed in the beginning. A minor variation is not at all a reason to
worry.
7. Avoid thinking too much about a questionThinking
about the questions before you attempt them is essential; but not to
such an extent that you waste a lot of time on one question.
Also
you need not write a very lengthy answer to a question just because the
question is easy and you know it very well. Remember, you need to just
answer the question and nothing more. Any over-attempt will be a mere
waste of time.